Page 28 - MATINF Nr. 3

Probleme de calcul integral. Inegalit˘ati integrale II
,

Florin St˘anescu 1

ˆ
In acest articol vom prezenta Inegalitatea Cauchy-Buniakovski-Schwarz, ˆın form˘a integral˘a,
dup˘a care vor urma o serie de aplicat , ii ale acestei inegalit˘at , i.

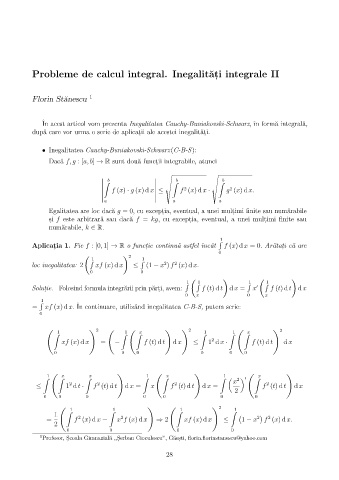
• Inegalitatea Cauchy-Buniakovski-Schwarz(C-B-S):

Dac˘a f, g : [a, b] → R sunt dou˘a funct , ii integrabile, atunci

Õ Õ

b b b
Z Z Z

f (x) · g (x) d x ≤ f (x) d x · 2
2
g (x) d x.

a a a
Egalitatea are loc dac˘a g = 0, cu except , ia, eventual, a unei mult , imi finite sau num˘arabile
s , i f este arbitrar˘a sau dac˘a f = kg, cu except , ia, eventual, a unei mult , imi finite sau
num˘arabile, k ∈ R.
1
R
Aplicat , ia 1. Fie f : [0, 1] → R o funct , ie continu˘a astfel ˆıncˆat f (x) d x = 0. Ar˘atat , i c˘a are
0
Ç å 2
1 1
R R
2
2
loc inegalitatea: 2 xf (x) d x ≤ (1 − x ) f (x) d x.
0 0
Ç å Ç å
1 1 1 1
R R R R
Solut ,ie. Folosind formula integr˘arii prin p˘art , i, avem: f (t) d t d x = x 0 f (t) d t d x
0 x 0 x
1
ˆ
R
= xf (x) d x. In continuare, utilizˆand inegalitatea C-B-S, putem scrie:
0
2 2
Ñ é Ñ Ñ é é Ñ é 2
1 1 x 1 1 x
Z Z Z Z Z Z
2
xf (x) d x = − f (t) d t d x ≤ 1 d x · f (t) d t d x
0 0 0 0 0 0

1 Ñ x x é 1 Ñ x é 1 Ñ x é
Z Z Z Z Z Z Å 2 ã 0 Z
x
2
2
2
2
≤ 1 d t · f (t) d t d x = x f (t) d t d x = f (t) d t d x
2
0 0 0 0 0 0 0
2
Ñ é Ñ é
1 1 1 1
Z Z Z Z
1
2
2
2
= f (x) d x − x f (x) d x ⇒ 2 xf (x) d x ≤ 1 − x 2 f (x) d x.
2
0 0 0 0
1
Profesor, S , coala Gimnazial˘a ,,S , erban Cioculescu”, G˘aes , ti, florin.florinstanescu@yahoo.com
28