Page 20 - MATINF Nr. 9-10

20 E.A. Jos´ Garc´ıa
e

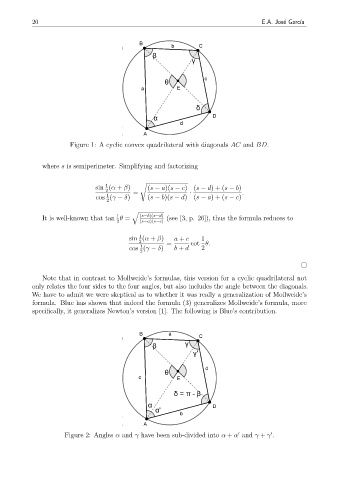
Figure 1: A cyclic convex quadrilateral with diagonals AC and BD.

where s is semiperimeter. Simplifying and factorizing

1
sin (α + β) (s − a)(s − c) (s − d) + (s − b)
2 = · .
1
cos (γ − δ) (s − b)(s − d) (s − a) + (s − c)
2
q
1
It is well-known that tan θ = (s−b)(s−d) (see [3, p. 26]), thus the formula reduces to
2 (s−a)(s−c)
1
sin (α + β) a + c 1
2 = cot θ.
1
cos (γ − δ) b + d 2
2

Note that in contrast to Mollweide’s formulas, this version for a cyclic quadrilateral not
only relates the four sides to the four angles, but also includes the angle between the diagonals.
We have to admit we were skeptical as to whether it was really a generalization of Mollweide’s
formula. Blue has shown that indeed the formula (3) generalizes Mollweide’s formula, more
specifically, it generalizes Newton’s version [1]. The following is Blue’s contribution.

0
0
Figure 2: Angles α and γ have been sub-divided into α + α and γ + γ .