Page 9 - MATINF Nr. 6

Two Identities and their Consequences 9

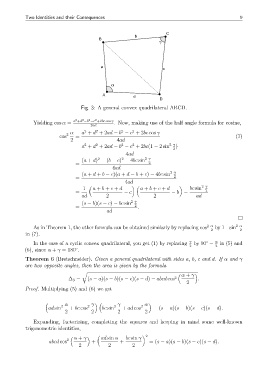
Fig. 3: A general convex quadrilateral ABCD.

2
2
2
2
Yielding cos α = a +d −b −c +2bc cos γ . Now, making use of the half angle formula for cosine,
2ad
2
2
2
2
α a + d + 2ad − b − c + 2bc cos γ
cos 2 = (7)
2 4ad
2
2
2
2
a + d + 2ad − b − c + 2bc(1 − 2 sin 2 γ )
= 2
4ad
2
2
(a + d) − (b − c) − 4bc sin 2 γ
= 2
4ad
(a + d + b − c)(a + d − b + c) − 4bc sin 2 γ
= 2
4ad
 ‹  ‹ 2 γ
1 a + b + c + d a + b + c + d bc sin
= − c − b − 2
ad 2 2 ad
(s − b)(s − c) − bc sin 2 γ
= 2 .
ad

As in Theorem 1, the other formula can be obtained similarly by replacing cos 2 α by 1−sin 2 α
2 2
in (7).
◦
In the case of a cyclic convex quadrilateral, you get (1) by replacing γ 2 by 90 − α in (5) and
2
◦
(6), since α + γ = 180 .
Theorem 6 (Bretschneider). Given a general quadrilateral with sides a, b, c and d. If α and γ
are two opposite angles, then the area is given by the formula
É
α + γ

∆ 3 = (s − a)(s − b)(s − c)(s − d) − abcd cos 2 .
2
Proof. Multiplying (5) and (6) we get
α γ γ α

ad sin 2 + bc cos 2 bc sin 2 + ad cos 2 = (s − a)(s − b)(s − c)(s − d).
2 2 2 2
Expanding, factorizing, completing the squares and keeping in mind some well-known
trigonometric identities,

α + 㠁 ad sin α bc sin γ ‹ 2
abcd cos 2 + + = (s − a)(s − b)(s − c)(s − d).
2 2 2