Page 7 - MATINF Nr. 6

Two Identities and their Consequences 7

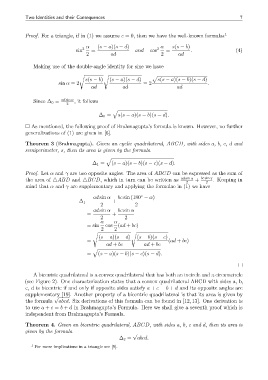
Proof. For a triangle, if in (1) we assume c = 0, then we have the well-known formulae 1

α (s − a)(s − d) α s(s − b)
sin 2 = and cos 2 = . (4)
2 ad 2 ad

Making use of the double-angle identity for sine we have

Ê Ê
p
s(s − b) (s − a)(s − d) s(s − a)(s − b)(s − d)
sin α = 2 = 2 .
ad ad ad

Since ∆ 0 = ad sin α , it follows
2
È
∆ 0 = s(s − a)(s − b)(s − d).
As mentioned, the following proof of Brahmagupta’s formula is known. However, no further
generalizations of (1) are given in [6].

Theorem 3 (Brahmagupta). Given an cyclic quadrilateral, ABCD, with sides a, b, c, d and
semiperimeter, s, then its area is given by the formula

È
∆ 1 = (s − a)(s − b)(s − c)(s − d).
Proof. Let α and γ are two opposite angles. The area of ABCD can be expressed as the sum of
the area of 4ABD and 4BCD, which in turn can be written as ad sin α + bc sin γ . Keeping in
2 2
mind that α and γ are supplementary and applying the formulae in (1) we have

◦
ad sin α bc sin (180 − α)
∆ 1 = +
2 2
ad sin α bc sin α
= +
2 2
α α
= sin cos (ad + bc)
2 2
Ê Ê
(s − a)(s − d) (s − b)(s − c)
= (ad + bc)
ad + bc ad + bc
È
= (s − a)(s − b)(s − c)(s − d).

A bicentric quadrilateral is a convex quadrilateral that has both an incircle and a circumcircle
(see Figure 2). One characterization states that a convex quadrilateral ABCD with sides a, b,
c, d is bicentric if and only if opposite sides satisfy a + c = b + d and its opposite angles are
supplementary [19]. Another property of a bicentric quadrilateral is that its area is given by
√
the formula abcd. Six derivations of this formula can be found in [12, 13]. One derivation is
to use a + c = b + d in Brahmagupta’s Formula. Here we shall give a seventh proof which is
independent from Brahmagupta’s Formula.

Theorem 4. Given an bicentric quadrilateral, ABCD, with sides a, b, c and d, then its area is
given by the formula √
∆ 2 = abcd.
1
For more implications in a triangle see [9].