Page 27 - MATINF Nr.2

A dynamic identity in a triangle and some applications 27

Remark 2. Using the last result, we can prove that in such triangles with b + c = 2a we have
0
a = d/2 = r, where r is the ABC-inradius, and the A-altitude is three times the ABC-inradius.
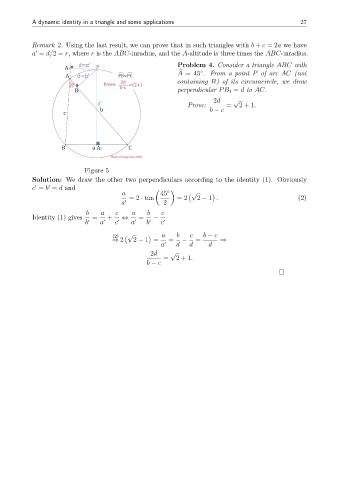
Problem 4. Consider a triangle ABC with
◦
A = 45 . From a point P of arc AC (not
“
containing B) of its circumcircle, we draw
perpendicular PB 1 = d to AC.

2d √
Prove: = 2 + 1.
b − c

Figure 5
Solution: We draw the other two perpendiculars according to the identity (1). Obviously
0
0
c = b = d and
a Ç 45 ◦ å Ä√ ä
= 2 · tan = 2 2 − 1 . (2)
a 0 2
b a c a b c
Identity (1) gives = + ⇔ = −
b 0 a 0 c 0 a 0 b 0 c 0

Ä√
(2) ä a b c b − c
⇒ 2 2 − 1 = = − = ⇒
a 0 d d d
2d √
= 2 + 1.
b − c